Impedance of Free Space (Part 3)

Back

In parts 1 and 2 of this series, we looked at the meaning of Electric and Magnetic fields, both static and when alternating at radio frequencies in free space, i.e. without any apparent intervening dielectric or ferro-magnetic materials. This article looks at what happens when these fields pass through a substance or even through empty space. But first an apology: The relationships dealt with in the following paragraph are dealt with very superficially.

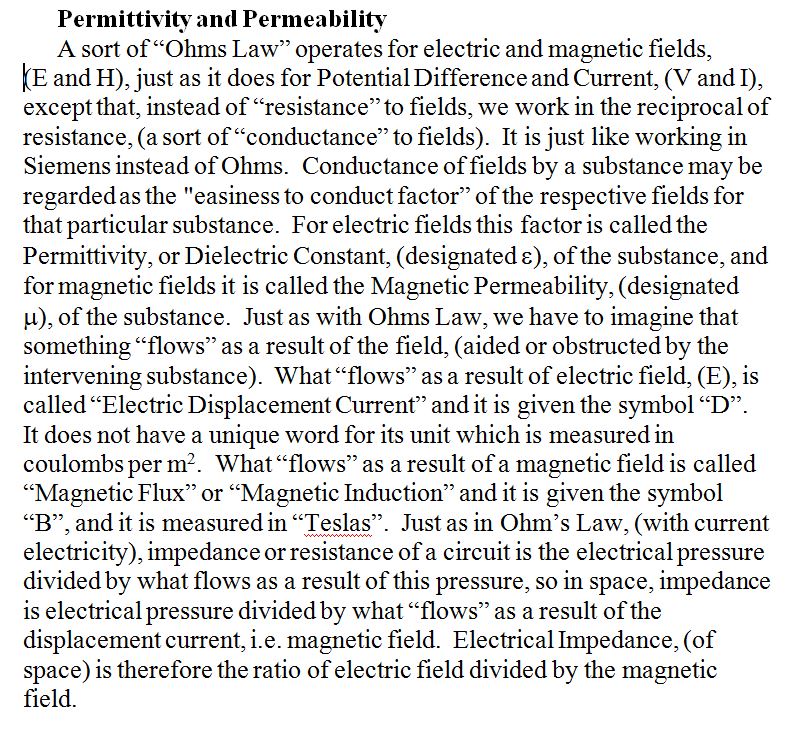
This is all rather theoretical, so let us get back to practicalities: In principle, we can determine the impedance of free space by measuring the electric and magnetic fields produced by a transmitter at some distant location and calculating their ratio, which gives us a figure of about 377 Ohms. (Actually it is never done this way because it can be derived more accurately from the velocity of light as we shall see later). Knowing the impedance of free space, we ought to be able to build a device out of suitable resistors, capacitors and inductors which would completely absorb the electromagnetic waves aimed at it; a sort of “space matched load”. (The ultimate in “stealth” technology?).. What would such a device look like? Here we once again run into hidden units.

Purely as an example of hidden units, which has little to do with the Impedance of Free Space, I shall consider “electrical resistivity”, since most Radio Amateurs who have designed some of their own equipment are fairly familiar with this, and have pondered the relative conductivity of silver, copper and aluminium etc.

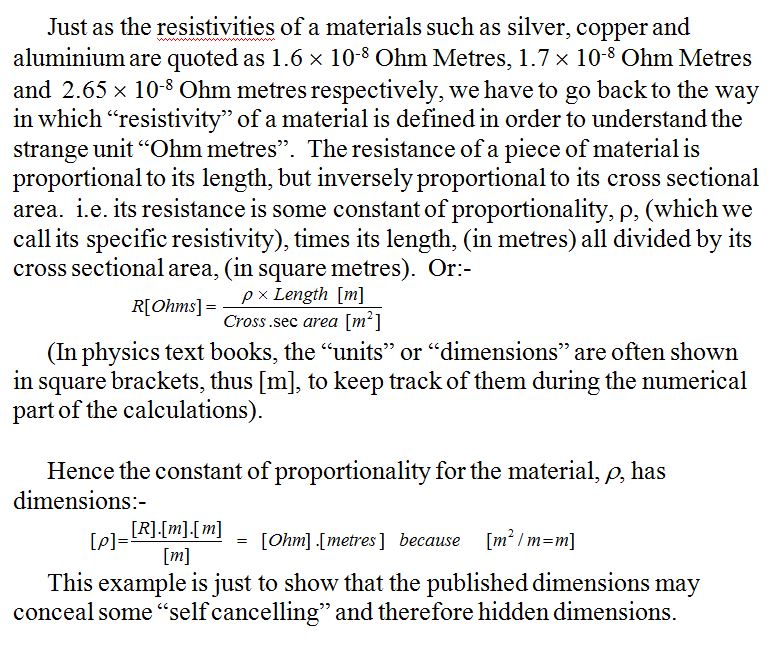
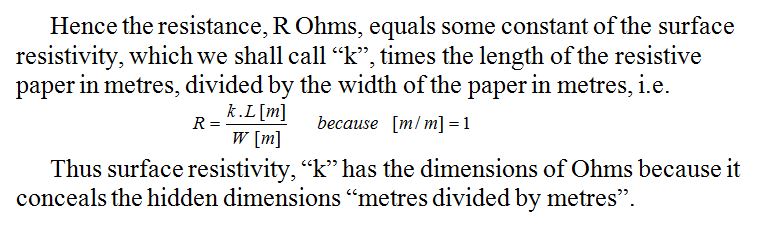
Now consider a square sheet of paper with an imperfectly conducting material deposited on it. (Paper which has been scribbled on all over with a graphite pencil is a typical example). Now, if a good conductor is deposited all along two of the opposite edges of the “resistive paper”, you could measure the resistance in ohms between these opposite edges. You would find of course, (for a given sample), that the resistance was proportional to the distance apart of these edges, i.e. the length, L, between them. You would also expect that the resistance would be inversely proportional to the width, W, of the conducting path as this can be imagined as lots of paths in parallel.

In this part, we have discovered the meaning of the impedance of free space and have proposed a possible application of this knowledge. In the next and last article, we shall look at the relationships between the various “space constants” and why some of them are known so accurately. We shall also look at the possibility of making a practical “reflectionless space matched load”.

Back

         John,   G0NVZ